 #60 The musical counterpart of the holistic
division: 384 = 192 + 192
#60 The musical counterpart of the holistic
division: 384 = 192 + 192| << Previous 1... 58 59 [60] 61 62 ...81 Next >> |
 #60 The musical counterpart of the holistic
division: 384 = 192 + 192
#60 The musical counterpart of the holistic
division: 384 = 192 + 192
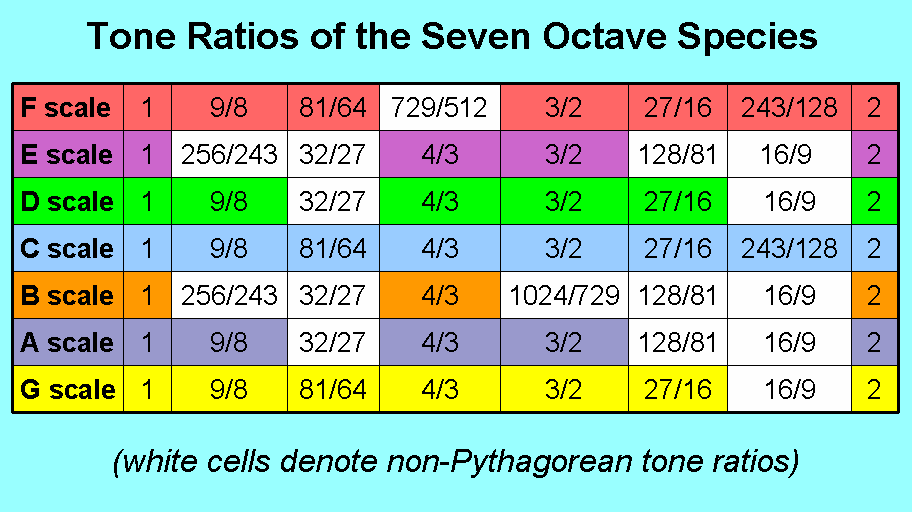
In #37 at The seven musical scales, it was shown that, between the tonic and octave of the seven types of musical scales:
which have 12 types of notes (six notes separated from their six inversions* by the vertical line shown below):
| 256/243 | 9/8 | 32/27 | 81/64 | 4/3 | 1024/729 |
│ |
729/512 | 3/2 | 128/81 | 27/16 | 16/9 | 243/128 |
there are 21 notes up to that with tone ratio 1024/729 and their 21 inversions. It was also shown that 189 rising intervals and 189 falling intervals exist between these notes. Including the tonic (1), the octave (2), the octave interval (2), the unit interval (1), the next lower octave (½) and the falling interval (½) of an octave, (189+3=192) rising & 192 falling intervals can be defined between the notes of the seven scales, each set of 192 intervals forming subsets of (21+3=24) intervals and 168 intervals. The analysis presented there (see the last row in the table of numbers of intervals and the last paragraph) shows that the 168 intervals divide into a set of 84, comprising 42 intervals up to 1024/729 and their 42 inversions and a set of 84 that comprises 63 unpaired intervals and the inversions of the 21 notes. #38 at The seven musical scales displays the geometrical representation of the (192+192) intervals in the first (6+6) enfolded polygons, each set of which has 192 intrinsic yods that include 24 corners of polygons (three shared with the outer Tree of Life) and 168 yods other than these corners. However, as we saw in #59, every example of true sacred geometry embodies the holistic parameter 384 and displays its various divisions. Hence, all these sacred geometries are representations in space of the complete composition of all possible rising and falling intervals between the notes making up the seven musical scales. Their characteristic divisions:
384 = 192 + 192 = 48 + 336,
192 = 24 + 168,
48 = 24 + 24,
24 = 3 + 21,
336 = 168 + 168,
and
168 = 84 + 84
have musical counterparts. In every case, the sacred geometry has two "halves" (usually mirror images of each other) that correspond to the distinction between the 192 rising intervals and the 192 falling intervals between the notes in the seven musical scales. Here, therefore, is clear, detailed evidence that sacred geometries and these scales share a universal pattern that is due not to coincidence but to the fact that they constitute truly holistic systems. See also #41 in The seven musical scales.
Some correspondences between the seven musical scales and the whorls of the
UPA/superstring
In string theory, the division: 24 = 3 + 21 manifests as follows:
"3" → the two large-scale, transverse dimensions of spinless strings in 26-dimensional space-time + the tenth dimension of its 11-dimensional hyperplane predicted by M-theory;
"21" → the six transverse, superstring compactified dimensions of this hyperplane + the 15 higher, transverse dimensions of 26-dimensional space-time;
"24" → the 24 transverse dimensions of a spinless string in 26-dimensional space-time.
The 90° phase difference between the left- and right-moving plane-wave oscillations in the two transverse, large-scale dimensions generates the circularly polarized standing wave that is the whorl of the UPA/subquark superstring. The 24 right-moving modes and the 24 left-moving modes of oscillation associated with the 24 E8 gauge charges spread along each whorl of the UPA correspond to the 24 rising note intervals and the 24 falling intervals discussed above. The 1680 1st-order spirillae in a whorl are their string manifestation. The 168 spirillae in an outer half-revolution of a whorl are the counterpart of the 168 rising intervals between the notes of the seven scales that were discussed earlier; the 168 spirillae in an inner half-revolution of a whorl correspond to their 168 falling intervals. This raises the possibility that the five revolutions of a whorl correspond to five successive octaves of the seven scales, the 168 rising intervals and the 168 falling intervals of their notes in an octave being repeated five times. The distinction between the two transverse, large-scale dimensions and the tenth spatial dimension predicted by M-theory, which separates the two space-time sheets containing ordinary and shadow matter superstrings, corresponds in the musical counterpart to the difference between the first and last notes of an octave (two actual notes) and the theoretical octave interval that they span. These correspond to what in the context of sacred geometry is labelled above as "3". The octave interval corresponds to the dimensional gap between the two space-time sheets predicted by E8×E8 heterotic superstring theory, whilst the 21 transverse, compactified dimensions other than the one separating the two space-time sheets correspond to the 21 notes in the seven scales up to that with tone ratio 1024/729, which are mirrored by their 21 inversions. Notice that these 21 notes comprise the six types of notes up to this one:
| 256/243 | 9/8 | 32/27 | 81/64 | 4/3 | 1024/729 |
and 15 repetitions of them. Compare this with the six compactified dimensions predicted by superstring theory and the 15 extra, compactified dimensions beyond 11-d space-time predicted by the application of quantum mechanics to spinless strings. The former are analogous to the six types of notes in the seven musical scales up to the one with tone ratio 1024/729, whilst the 15 dimensions predicted by bosonic string theory to exist beyond the tenth dimension required by M-theory correspond to the 15 further instances of these notes in the seven scales.
#37 at The seven musical scales provides evidence that it is not by chance that the number 21 of EHYEH (Hebrew: אהיה, AHIH), the Godname of Kether, is the number of notes above the tonic up to 1024/729, as well as the number of their inversions. It indicates that the gematria number values of the Hebrew letters of this Godname are the numbers of the six notes, as well as the numbers of their inversions, whilst the letter values Y = 10 & H = 5 of YAH (Hebrew: יה, YH), the Godname of Chokmah with number value 15, denote the numbers of repeated instances of these six notes, as well as the numbers of repetitions of their inversions.
|
AHIH = 21 |
YH = 15 |
||||
|
Notes |
Inversions |
Repeated notes |
Repeated inversions |
||
| A = 1 → 1×(1024/729) |
→ 1×(729/512) |
0×(1024/729) | 0×(729/512) | ||
| H = 5 → 5×(9/8) |
→ 5×(16/9) |
4×(9/8) | 4×(16/9) | ||
| I = 10 → 4×(32/27) + 6×(4/3) |
→ 4×(27/16) + 6×(3/2) |
3×(32/27) | 3×(27/16) | ||
| H = 5 → 2×(256/243) + 3×(81/64) |
→ 2×(243/128) + 3×(128/81) |
2×(81/64) | 2×(128/81) | ||
| Total = 21 | Total = 21 | 1×(256/243) | 1×(243/128) | ||
|
Subtotal = |
10 = Y |
Subtotal = |
10 = Y | ||
|
H = 5 → |
5×(4/3) |
H = 5 → |
5×(3/2) |
||
|
Total = |
15 |
Total = |
15 | ||
H = 5 measures the number of perfect fourths that are repeated in the seven musical scales and Y = 10 measures the ten repetitions of the other notes (similarly for their inversions numbered in the last column of the table). By counting the coloured cells in the table of tone ratios of notes shown above, it can be verified that the number value 26 of YAHWEH (יהוה), the complete Godname of Chokmah, is the number of notes between the tonic and octave that are found in the Pythagorean musical scale (C scale). It is remarkable that the 6:15 division of the Godname number 21 manifests in both the UPA/superstring and the seven musical scales, whilst the Godname number 26 appears as the number of dimensions of the space-time of spinless strings and as the number of Pythagorean notes in the seven scales. However, it should not be regarded as surprising. This is because both superstrings and the seven musical scales are examples of holistic systems, which means that they are mathematically prescribed by these (and other) Godnames, with properties that are quantified by their letter values. Article 16 analyzes in depth the composition of the intervals between the notes of the seven octave species and discusses its correspondence with octonion algebra and superstrings.
| << Previous 1... 58 59 [60] 61 62 ...81 Next >> |