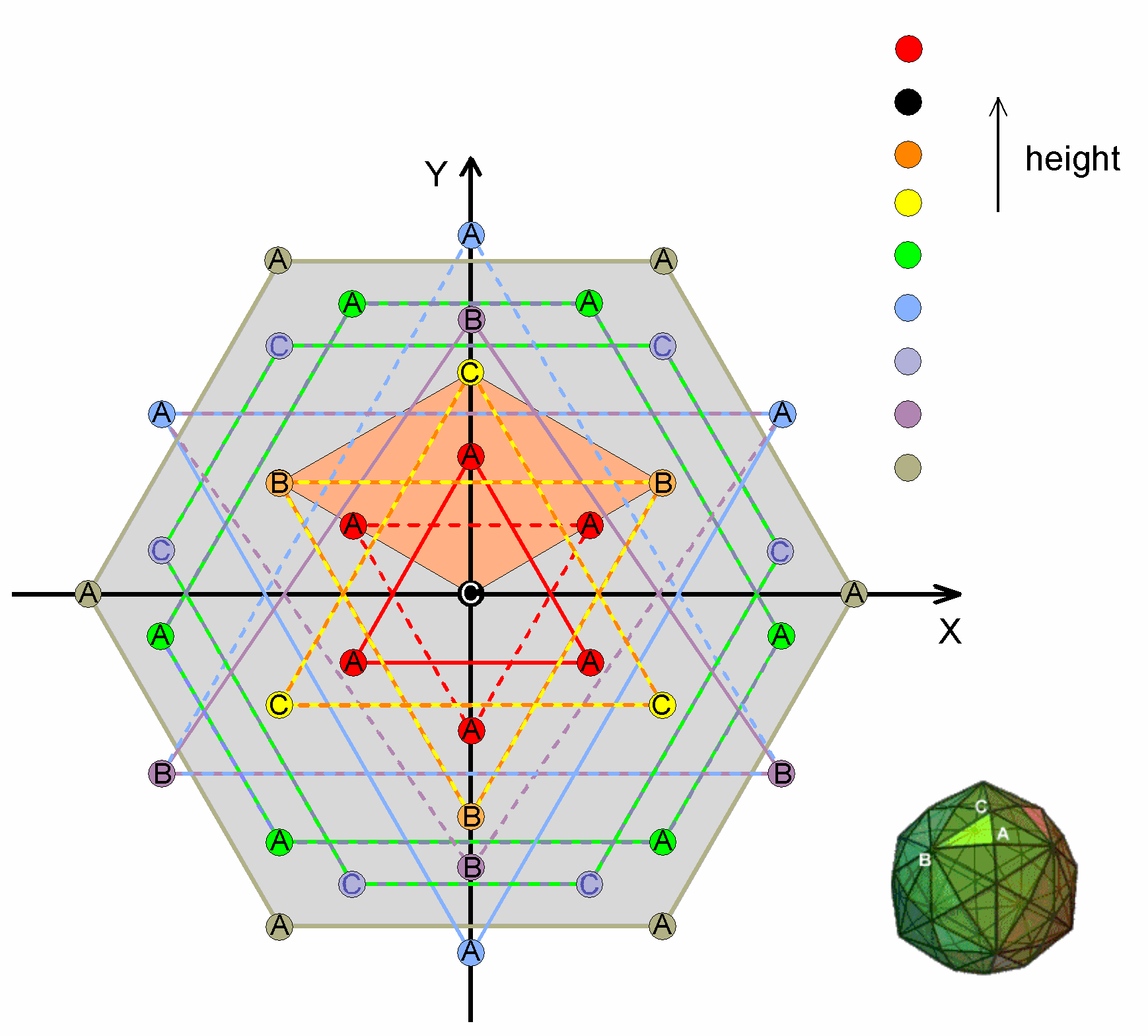
When the axis of the disdyakis triacontahedron passes through two diametrically opposite C vertices (vertices of a dodecahedron), the 60 vertices surrounding it are corners of 15 polygons. This shows how the Godname YAH of Chokmah with number value 15 prescribes the disdyakis triacontahedron. Starting from the XY plane, the eight polygons in half the polyhedron are:

1. hexagon with six khaki A vertices;
2. triangle with three violet B vertices;
3. 6-sided polygon with six grey C vertices;
4. triangle with three blue A vertices;
5. 6-sided polygon with six green A vertices;
6. triangle with three yellow C vertices;
7. triangle with three orange B vertices;
8. triangle with three red A vertices.

The seven polygons in the lower half of the polyhedron have edges indicated by dashed lines. The two central, black C vertices are not the apex and nadir of the polyhedron because three red A vertices are, respectively, above and below them, as indicated in the diagram showing the height of vertices above the XY plane (their mirror images below this plane are not shown). The graph displays the Golden Rhombus formed by two B vertices and two C vertices, the black one of which is the "North pole", although it is not the highest point of the disdyakis triacontahedron when the latter has the orientation shown in the diagram.

 

<< Previous    1...   9  10  [11]  12  13  ...15    Next >>

Home