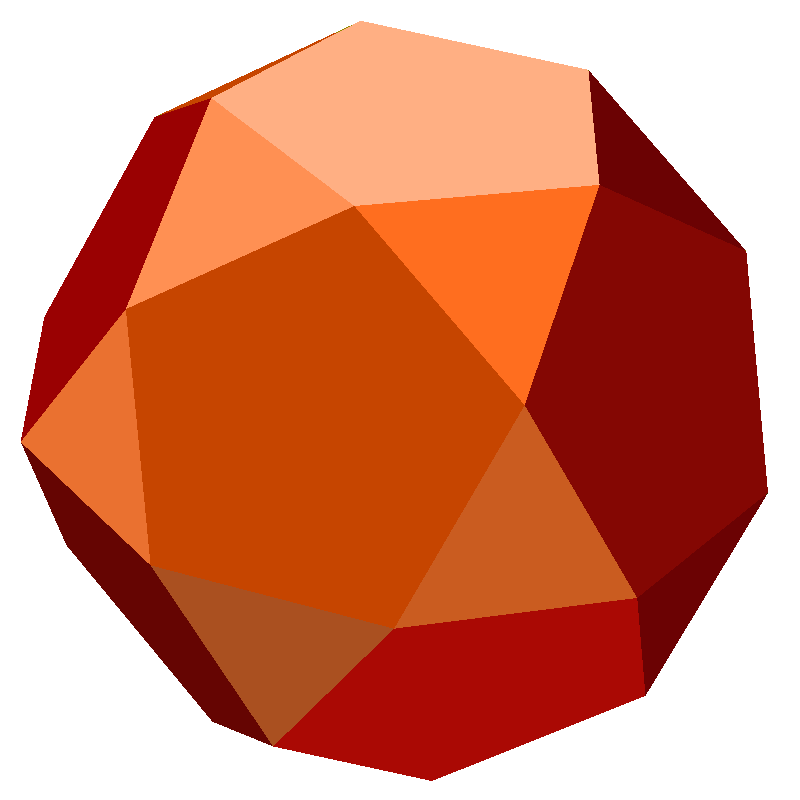
Icosidodecahedron

               480 corners
               1680 red yods
               1680 blue yods

            Total = 3840 yods

The icosidodecahedron, whose 30 vertices coincide with some of the vertices of the disdyakis triacontahedron, was excluded from the discussion above because it plays a special role in defining the superstring structural parameter 3360. Having 20 triangular faces, 12 pentagonal faces & 60 edges, it has 480 hexagonal yods in its 32 faces — just as the rhombic triacontahedron does in its 30 faces. This means that the 29 Platonic, Archimedean & Catalan solids formed by vertices of the disdyakis triacontahedron have (3360+480=3840) hexagonal yods. This is the number of yods in 384 tetractyses. The division:

3840 = 480 + 3360

is the counterpart of the 48 lines & broken lines in the eight diagonal hexagrams of the I Ching table and the 336 lines & broken lines in its 56 off-diagonal hexagrams. The 168 lines & broken lines in each diagonal half of the 8×8 array of hexagrams correspond to the 1680 hexagonal yods in either the 21 Platonic solids or the seven other polyhedra (alternatively, they correspond to the 1680 hexagonal yods in each half of all 28 Platonic & Catalan solids that can fit inside the disdyakis triacontahedron). Indeed, the 48:336 division is characteristic of all holistic systems embodying sacred geometry, as many research articles at this website illustrate (see also the discussion under "192 = 24 + 168" at The holistic pattern). Its manifestation in the disdyakis triacontahedron is yet more evidence of the holistic nature of this polyhedron, which is shown in Article 23 to be the outer form of the Polyhedral Tree of Life (see also here. This is confirmed by the fact that the Decad, called "All complete" and "Cosmos" by the Pythagoreans, determines the number of polyhedra that can fit the disdyakis triacontahedron because 29 is the tenth prime number.

The counterparts of this 480:3360 division in the inner Tree of Life are the 3360 red & blue yods other than polygonal corners in the 120 polygons of the first six types enfolded in the 10-tree shown opposite and the 480 corners (black circles) of these polygons that are intrinsic to them in the sense that none of them are shared with polygons enfolded in the next higher Tree of Life. Notice that there are no black circles at the topmost corners of the two hexagons enfolded in the tenth Tree of Life, because these corners coincide with the lowest corners of the two hexagons (not shown) enfolded in the eleventh Tree of Life. The two sets of the first six polygons constitute a holistic set in themselves, being prescribed by the ten Godnames (e.g., they have 50 corners, where 50 is the gematria number value of ELOHIM, the Godname of Binah; see Article 4 for how other Godnames prescribe them). The correspondence between polyhedra and polygons is shown below:

1680 coloured yods (840 red, 840 blue) in the 60 polygons enfolded on one side of the 10-tree → 1680 hexagonal yods in the faces of the 21 Platonic solids.
1680 coloured yods (840 red, 840 blue) in the 60 polygons enfolded in the other side of the 10-tree → 1680 hexagonal yods in the faces of the 7 Catalan solids.
480 corners (white circles) of the 120 polygons → 480 hexagonal yods in the faces of the icosidodecahedron.

As each Tree of Life is a representation of a Sephirah of the Tree of Life, this correspondence demonstrates unequivocally the Tree of Life nature of the disdyakis triacontahedron.

It may be asked why, as they both have the same number of hexagonal yods, the icosidodecahedron, rather than its dual, the rhombic triacontahedron, should be associated with the corners of the 120 enfolded polygons. At the time of writing, the only argument that can be offered is that the former is an Archimedean solid, whereas the 28 other polyhedra that can be accommodated in the disdyakis triacontahedron are either Platonic or Catalan solids. This mirrors the fundamental distinction between yods at corners of the polygons and their other yods. Hopefully, a more rigorous reason than this analogy will appear that eliminates the ambiguity of assignment.

Whether it is embodied in the polyhedrons that can fit into the disdyakis triacontahedron or in the inner form of the Tree of Life, the number 3360 is the number of circular turns in each of the five revolutions of the ten whorls of the UPA. The 240 gauge charges of the gauge symmetry group E₈ are spread along the whorls, 48 charges per 360° revolution of all 10 whorls and 70 turns per gauge charge. These charges are the sources of 10-dimensional gauge fields. Therefore, their 48 associated gauge fields have 480 space-time components. The inner Tree of Life representation of the space-time structure of the E₈×E₈ heterotic superstring is telling us that the 480 corners of the 120 enfolded polygons denote the 480 space-time components of the 48 gauge charges spread along each revolution of the ten whorls and that the 3360 remaining yods denote the 3360 circularly polarized oscillations of the whorls that "carry," so to speak, these charges. As 48 is both the number value of Kokab, the Mundane Chakra of Hod, and the number of corners of the seven separate polygons making up each half of the inner Tree of Life, this number is a defining parameter of holistic systems that embody the divine blueprint. Each revolution of the ten whorls, along which the 48 gauge charges are spread, should be seen as constituting in itself a whole that is repeated five times (i.e., in the five revolutions around the axis of spin of the UPA/subquark superstring) in order to complete closure of the curves of the heterotic superstring.

See also #15 at Wonders of correspondences.